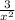Question

Help !!!
See question in image.
Please show workings .
​

Answer 1

Given a function y=f(x) its first derivative – the rate of change of y with respect to x – is defined by: dydx=limh→0[f(x+h)−f(x)h]. Finding the derivative of a function by computing this limit is known as differentiation from first principles

and put the formula answer will be there

Answer 2

see explanation

Explanation:

Given f(x) then the derivative f'(x) is

f'(x) = lim( h tends to 0 )
`(f(x+h)-f(x))/(h)`

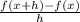
= lim( h to 0 )
`((3)/(x+h)-(3)/(x) )/(h)`

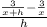
= lim( h to 0 )
`(3x-3(x+h))/(hx(x+h))`

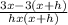
= ( lim h to 0 )
`(3x-3x-3h)/(hx(x+h))`

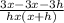
= lim( h to 0 )
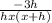`(-3h)/(hx(x+h))` ← cancel h on numerator/ denominator

= lim( h to 0 )
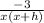`(-3)/(x(x+h))` ← let h go to zero, then

f'(x) = -
`(3)/(x^2)`