Question

Alicia has 6 paintings she wants to hang side by side on her wall.

A) In how many ways can she arrange all 6 paintings? Show your work.
B) If she only wants to display 4 of the paintings, in how many ways can she choose the paintings she wishes to display? Show your work.
C) In how many ways can she arrange 3 out of the 6 paintings? Show your work.

Answer 1

A) 720 ways

B) 15 ways

C) 6 ways

Explanation:

A) To find the number of ways Alicia can arranger her 6 paintings, we will find factorial of 6 by multiplying all of the positive integers equal to or less than that number i.e. 6 to get:

6! = 6 * 5 * 4 * 3 * 2 * 1 = 720

Alicia can arrange her paintings in 720 ways.

B) We use the following formula (when order is not important) to find the number of permutations of n objects taken r at a time:

`P(n, r) = (n!)/(r!(n-r)!)`

`= (6!)/(4!(6-4)!)  = 15`

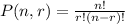
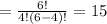
Therefore, Alicia can choose any 4 of her paintings in 15 ways.

C) Number of ways Alicia can arrange 3 out of 6 paintings = 3! = 3*2*1 = 6 ways