Question

You have two rectangles, rectangle ABCD and EFGH. The length of AB is x meters. The length of CD is 12 meters. The length of EF is 16 meters. The length of FG is x - 2 meters. You want to find the value of x so that the rectangles have the same area.

Answer 1

Let the area of the rectangle ABCD be denoted by
`A_1`.

Therefore,
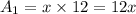`A_1=x* 12=12x`........(Equation 1) [Reason: Area of any rectangle is the product of it's length and breadth]

Likewise, let the area of the rectangle EFGH be denoted by
`A_2`

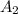
Therefore,
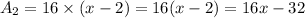`A_2=16 * (x-2)=16(x-2)=16x-32`....(Equation 2) [Reason: Area of any rectangle is the product of it's length and breadth]

Since the area of the two rectangles are equal, we will have:

`A_1=A_2`

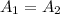
Therefore, equating the two equations (Equation 1 and Equation 2) we get:

`12x=16x-32`

`12x-16x=-32`

`-4x=-32`

`x=(-32)/(-4)=8`

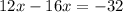
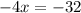
Thus, the value of x such that the rectangles have the same area is 8 meters.