Question

What are the discontinuities of the function f(x) = the quantity x squared minus 36 over the quantity 4x minus 24?

Answer 1

x^2 - 36

----------- = (x - 6)(x + 6) / 4(x - 6)

4x - 24

When x = 6 the denominator = 0 so the discontinuity is x = 6.

Answer 2

Discontinuities of the function is x = 6 .

Explanation:

Given : f(x) = the quantity x squared minus 36 over the quantity 4x minus 24

To find : What are the discontinuities of the function .

Solution : We have given

f(x) =
`(x^(2)- 36 )/(4x - 24)`.

On factoring
`x^(2)- 36 = (x+6) (x -6)`

4x - 24 = 4 ( x -6 ).

Then , f(x) =
`((x-6) (x +6) )/(4(x -6))`.

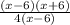f(x) =
`((x-6)(x +6) )/(4(x -6))`.

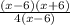A point of discontinuity occurs when a number is both a zero of the numerator and denominator.

Hence , at x = 6 both numerator and denominator is zero.

Therefore, Discontinuities of the function is x = 6 .