2 Answers

Simonwo · Answer 1 · 2019-10-22T12:21:22+0000

Answer:

Explanation:

And required prove is

and ( given)

And, (given)

and are right angle triangle.( perpendicular lines form 90° angle)

So,

( Common angle property )

( ASA postulate)

Tsewang · Answer 2 · 2019-10-25T19:11:58+0000

Answer: We can fill the columns with help of below explanation.

Explanation: Here, we have a figure in which we have two triangles ADC and BEC.

And required prove is
$\triangle ADC\cong\triangle BEC$

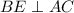$BE\perp AC$ and
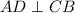$AD\perp CB$ ( given)

And,
$CB\cong CA$ (given)

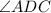$\angle ADC$ and
$\angle BEC$ are right angle triangle.( perpendicular lines form 90° angle)

So,
$\angle ADC \cong \angle BEC$

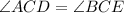$\angle ACD=\angle BCE$ ( Common angle property )

$\triangle ADC\cong\triangle BEC$ ( ASA postulate)

Please log in or register to add a comment.