Question

An empty airplane with a mass of 200,000 kg must have a speed of 82 m/s to achieve takeoff. Once it is fully loaded, the airplane has a mass of 350,000 kg. It has 3200 m of runway. a) How much force is needed to get the full airplane safely in the air?

b) How much runway would the empty airplane use if its engines generated the same force?

Answer 1

a) A force of 367718.75 newtons is needed to get the full airplane safely in the air.

b) The empty airplane would need a runway of 1828.571 meters.

Step-by-step explanation:

a) This problem can be solved by using the Work-Energy Theorem, which states that work needed by the airplane to get minimum speed is equal to its change in translational kinetic energy, both measured in joules. The resulting formula is presented below:

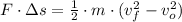`F\cdot \Delta s = (1)/(2)\cdot m \cdot (v_(f)^(2)-v_(o)^(2))` (1)

Where:

`F` - Minimum net force, measured in newtons.

`\Delta s` - Runway length, measured in meters.

`m` - Mass of the airplane, measured in kilograms.

`v_(o)`,
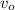`v_(f)` - Initial and final speeds of the airplane, measured in meters per second.

If we know that
`m = 350000\,kg`,
`v_(o) = 0\,(m)/(s)`,
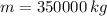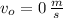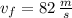`v_(f) = 82\,(m)/(s)` and
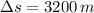`\Delta s = 3200\,m`, then the minimum net force needed by the airplane to get itself safely in the air:

`F = (m\cdot (v_(f)^(2)-v_(o)^(2)))/(2\cdot \Delta s)`

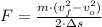
`F = ((350000\,kg)\cdot \left[\left(82\,(m)/(s) \right)^(2)-\left(0\,(m)/(s) \right)^(2)\right])/(2\cdot (3200\,m))`

`F = 367718.75\,N`

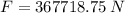
A force of 367718.75 newtons is needed to get the full airplane safely in the air.

b) If we know that
`m = 200000\,kg`,
`v_(o) = 0\,(m)/(s)`,
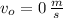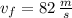`v_(f) = 82\,(m)/(s)` and
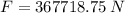`F = 367718.75\,N`, then the length of the runway is:

`\Delta s = (m\cdot (v_(f)^(2)-v_(o)^(2)))/(2\cdot F)`

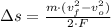
`\Delta s = ((200000\,kg)\cdot \left[\left(82\,(m)/(s) \right)^(2)-\left(0\,(m)/(s) \right)^(2)\right])/(2\cdot (367718.75\,N))`

`\Delta s = 1828.571\,m`

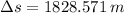
The empty airplane would need a runway of 1828.571 meters.