Answer:
In this type of question;
The transformation is that how you move same unit together or one side.
Multiplication Property of equality states that you multiply both sides of an equation by the same number.
(1)
Solve:
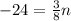
Using multiplication property of equality.
Multiply both sides of an equation by
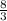 ; we have
; we have
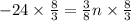
Simplify:

or
n = -64.
Therefore, in this option B is correct.
(2)
Solve:
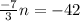
Using multiplication property of equality.
Multiply both sides of an equation by
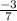 ; we have
; we have

Simplify:

or
n =18.
Therefore, the option A is correct in this question.
(3)
Solve:
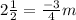
or we can write this as;

Using multiplication property of equality.
Multiply both sides of an equation by
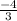 ; we have
; we have
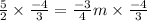
Simplify:
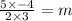
or
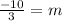
or

therefore, the options B is correct in this question