Question

Vector addition of a=8km[E] and b=13km[E]

Answer 1

The answer to the question is 21 km towards east.

CALCULATION:

Let us consider two vectors
`\vec A \ and\ \vec B`

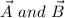
As per the question,
`\vec A=8\ km\ towards\ east`

`\vec B=\ 13\ km\ towards\ east`

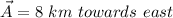
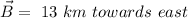
It is obvious that two vectors are parallel to each other. Hence, the angle between them is zero i.e
`\theta\ = 0^0`

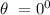
We are asked to calculate the resultant .

As per parallelogram law of vector addition, the magnitude of resultant will be-

`R=\sqrt{A^(2) +B^(2) +2ABcos\theta}`

`=√(8^2+13^2+2* 8*13 cos0)`

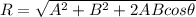
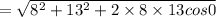
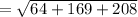`=√(64+169+208)` [∵ cos0 = 1]

`=√(441)\ km`

`=\ 21\ km`

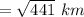
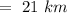
The direction of the resultant will be also towards east as the two vectors are parallel to each other.

Hence, the vector addition of two vectors are 21 km towards east.