Answer:
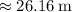
Explanation:
Let
 be the height of the tree. In reference to the tree and bird, a right triangle is formed. We can write the following trig function for that right triangle:
be the height of the tree. In reference to the tree and bird, a right triangle is formed. We can write the following trig function for that right triangle:
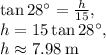 .
.
Now we can use this to find the hypotenuse of this triangle (the distance between the cat and the bird):
Let the distance between the cat and bird be
 . Then,
. Then,
 . This is one side of the triangle that is formed by the cat, bird, and mouse.
. This is one side of the triangle that is formed by the cat, bird, and mouse.
The right triangle formed by the tree, cat, and mouse shares the height
 with the other right triangle, as we found earlier. Let
with the other right triangle, as we found earlier. Let
 represent the hypotenuse of this triangle or the distance between the cat and mouse. We then have the following trig function:
represent the hypotenuse of this triangle or the distance between the cat and mouse. We then have the following trig function:
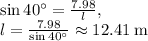 . This is another side of the triangle that is formed by the cat, bird, and mouse.
. This is another side of the triangle that is formed by the cat, bird, and mouse.
The triangle that is formed by the cat, bird, and mouse has shares an angle of
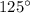 with the base of the auxiliary pyramid. The other angles, however, are not shared.
with the base of the auxiliary pyramid. The other angles, however, are not shared.
Since we have two sides and the angle between these two sides, we can use the Law of Cosines to find the other side (the distance between the mouse and bird). The Law of Cosines is given by:
 , where
, where
 is the distance between the bird and the mouse and the other variables are defined above.
is the distance between the bird and the mouse and the other variables are defined above.
Plugging in values, we get:
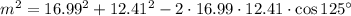
Solving, we get:
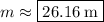 .
.