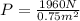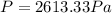Question

1. Suppose a tank filled with water has a liquid column with a height of 10 meters. If the area is 2 square meters (m²), what's the force of gravity acting on the column of water?

2. If a total force exerted by water in a container with a bottom area of 3 square meters is 900 newtons, what is the water pressure at the bottom of the container?
3. A tank with a flat bottom is filled with water to a height of 7.5 meters. What's the pressure at any point at the bottom of the tank? (You can ignore atmospheric pressure in your calculations.)
4. In a tank full of water, the pressure on a surface 2 meters below the water level is 1.5 kPa. What's the pressure on a surface 6 meters below the water level?
5. A piston above a liquid in a closed container has an area of 0.75 m², and the piston carries a load of 200 kg. What will be the external pressure on the upper surface of the liquid?

Answer 1

Answer:1. F = Ahdg

F = 2 m2 × 10 m × 1,000 kg/m3 × 9.8 m/s2

F = 20 × 1,000 × 9.8

F = 20,000 × 9.8

F = 196,000 N

2. P=FA

P=900N3m2

P = 300 Pa

This could also be written as 0.300 kPa. To convert from pascals to kilopascals, simply divide by 1,000.

3. P = hdg

P = 7.5

P = 7.5 m × 1,000 kg/m2 × 9.8 m/s2

P = 73,500 pascals

This could also be written as 73.5 kPa. To convert from pascals to kilopascals, simply divide by 1,000.

4. The height of the water column is 3 times higher at 6 meters below the water level. Multiply the pressure at 2 meters by 3 to calculate the pressure at 6 meters.

P = 3 × 1.5 kPa

P = 4.5 kPa

5. P=mgA

P=200kg×9.8m/s20.75m/s2

P=1,9600.75

P = 2,613 Pa or 2.613 kPa

Step-by-step explanation:

penn

Answer 2

Answers:

1. Firstly, we have to define that Pressure
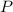`P` is Force applied
`F` per unit area
`A`. It is mathematically expressed as follows:

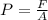`P=(F)/(A)` (1)

The unit of P is Pascal (Pa) which is equivalent to
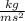`(kg)/(ms^(2))` and also equivalent to
`(N)/(m^(2) )`

There is also another expression of the Pressure in which it is dependent on the density
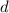`d` of the liquid, the height
`h` of the container and the gravity force
`g`:

`P=d*h*g` (2)

In this problem the liquid is water, and its known density is approximately:

`d=1000kg/m^(3)`

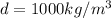
So, we have to substitute the values in equation (2) to obtain the pressure (Being careful with the units):

`P=1000(kg)/(m^(3))*10m*9.8(m)/(s^(2))`

`P=98000Pa`

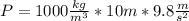
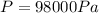
Then, we have to substitute this value in equation (1) and clear
`F`:

`F=P*A`

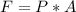
Finally:

`F=196000N`

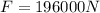
2. For this problem, we will use equation (1) to find the Pressure. We already know the area
`A` and the force exerted by water in the container
`F`:

`P=(F)/(A)=(900N)/(3m^(2))`

`P=300Pa`

3. In this case, equation (2) is the perfect way to find the hydrostatic pressure at any point at the bottom of the tank (be careful with the units):

`P=d*h*g`

`P=1000(kg)/(m^(3))*7.5m*9.8(m)/(s^(2))`

`P=73500Pa`

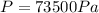
4. In this case, it's important to know that in fluids (in this case the water) the higher the fluid is, the lower the pressure. Then, if
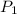`P_(1)` and
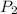`P_(2)` are the respective pressures at the heights
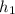`h_(1)` and
`h_(2)`, and knowing that the water density and the gravity force in this case are constants, we can use the following expression to solve this problem:

`P_(2)- P_(1) =d*g(h_(2)- h_(1))` (3)

Where:

`P_(1)=1.5 kPa` at
`h_(1)=2m`

Note that
`1kPa=1*1000 Pa`

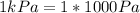
And
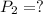`P_(2)=?` is unknown at a given height
`h_(2)=6m`

Then, we have to substitute the values in equation (3) to find
`P_(2)`:

`P_(2)-1500Pa=1000(kg)/(m^(3))*9.8(m)/(s^(2)) (6m-2m)`

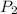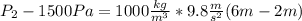
Finally:
`P_(2) =40700Pa`

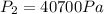
5. In this case we have the area
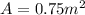`A=0.75m^(2)` and the mass of the piston
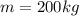`m=200kg`, and we need to know the pressure
`P`.

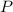We will use equation (1):

`P=(F)/(A)`

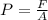
But, do you remember that above we stated that pressure is the force applied over an area?

Well, in this case we will use the following equation, in which the gravity force and the mass of a body are involved, to find
`F`:

`F=m*g=200kg*9.8(m)/(s^(2))`

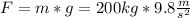
Then:

`F=1960N`

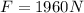
Now we can finally calculate
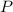`P`:

`P=(1960N)/(0.75m^(2))`

`P=2613.33Pa`