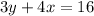ANSWER
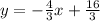
Or
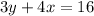
EXPLANATION
Let us find the gradient of the line:
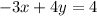 by rewriting it in the slope intercept form.
by rewriting it in the slope intercept form.
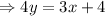
We divide through by 4 now;
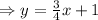
This is now in the form;
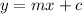
where
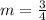 is he slope.
is he slope.
This implies that the slope of the line that is perpendicular to this line will be the negative reciprocal of
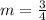 .
.
Thus the perpendicular line has slope,
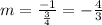 .
.
Let the perpendicular line have equation,
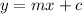
When we substitute the slope we have;
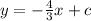
We substitute the point.
 to find c.
to find c.
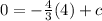
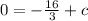
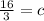
We substitute c to obtain;
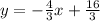
Or