Question

What mass of oxygen (O2) forms in a reaction that forms 15.90 g C6H12O6? (Molar mass of O2 = 32.00 g/mol; molar mass of C6H12O6 = 180.18 g/mol) 15.90 g C6H12O6 = g O2

Answer 1

The answer is: the mass of oxygen is 16.95 grams.

The overall balanced photosynthesis reaction:

6CO₂ + 6H₂O → C₆H₁₂O₆ + 6O₂.

m(C₆H₁₂O₆) = 15.90 g; mass of glucose.

n(C₆H₁₂O₆) = m(C₆H₁₂O₆) ÷ M(C₆H₁₂O₆).

n(C₆H₁₂O₆) = 15.9 g ÷ 180.18 g/mol.

n(C₆H₁₂O₆) = 0.088 mol; amount of glucose.

From chemical reaction: n(C₆H₁₂O₆) : n(O₂) = 1 : 6.

n(O₂) = 6 · 0.088 mol.

n(O₂) = 0.53 mol; amount of oxygen.

m(O₂) = 0.53 mol · 32.00 g/mol.

m(O₂) = 16.95 g; mass of oxygen.

Answer 2

Answer : The mass of
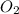`O_2` forms are 16.94 grams.

Explanation : Given,

Mass of
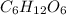`C_6H_(12)O_6` = 15.90 g

Molar mass of
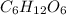`C_6H_(12)O_6` = 180.18 g/mole

Molar mass of
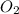`O_2` = 32.00 g/mole

The balanced chemical reaction will be:

`6CO_2(g)+6H_2O(g)\rightarrow C_6H_(12)O_6(s)+6O_2(g)`

First we have to calculate the moles of
`C_6H_(12)O_6`

`\text{ Moles of }C_6H_(12)O_6=\frac{\text{ Mass of }C_6H_(12)O_6}{\text{ Molar mass of }C_6H_(12)O_6}=(15.90g)/(180.18g/mole)=0.08824moles`

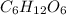
Now we have to calculate the moles of
`O_2`

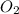
As, 1 mole of
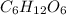`C_6H_(12)O_6` produced when 6 moles of oxygen produced

So, 0.08824 mole of
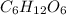`C_6H_(12)O_6` produced when
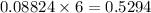`0.08824* 6=0.5294` moles of oxygen produced

Now we have to calculate the mass of
`O_2`

`\text{ Mass of }O_2=\text{ Moles of }O_2* \text{ Molar mass of }O_2`

`\text{ Mass of }O_2=(0.5294moles)* (32.00g/mole)=16.94g`

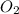
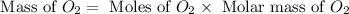
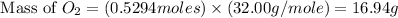
Therefore, the mass of
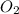`O_2` forms are 16.94 grams.