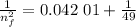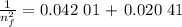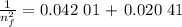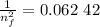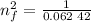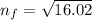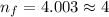Question

what electronic transition in a hydrogen atom starting from n=7 energy level will produce infrared radiation with energy of 55.1 kj/mol?

Answer 1

(n = 7) ⟶ (n = 4)

Explanation:

1. Convert the energy to joules per mole of electrons.

E = 55.1 × 1000 = 55 100 J/mol

2. Convert the energy to joules per electron

E = 55 100/(6.022 × 10²³)

E = 9.150 × 10⁻²⁰ J/electron

3. Use the Rydberg equation to calculate the transition

Rydberg's original formula was in terms of wavelengths, but we can rewrite it to have the units of energy. The formula then becomes

`\Delta E = R_{\text{H}} (( 1)/( n_(f)^(2)) - ( 1)/( n_(i)^(2)))`

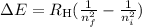
where

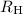`R_{\text{H}}` = the Rydberg constant = 2.178 × 10⁻¹⁸ J

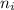`n_(i)` and
`n_(f)` are the initial and final energy levels.

`9.150 * 10^(-20) = 2.178 * 10^(-18)(( 1)/( n_(f)^(2)) - ( 1)/( 7^(2)))`

`(9.150 * 10^(-20) )/(2.178 * 10^(-18)) = ( 1)/( n_(f)^(2)) - ( 1)/( 49)`

`( 1)/( n_(f)^(2)) = \text{0.042 01} + (1 )/(49 )`

`( 1)/( n_(f)^(2)) = \text{0.042 01 + 0.020 41}`

`( 1)/( n_(f)^(2)) = \text{0.042 01 + 0.020 41}`

`( 1)/( n_(f)^(2)) = \text{0.062 42}`

`n_(f)^(2) = \frac{1 }{ \text{0.062 42}}`

`n_(f)^(2) = 16.02`

`n_(f) = √(16.02)`

`n_(f) = 4.003 \approx 4`