Question

The length of a rectangular painting is 3 inches longer than its width. If the diagonal is 15 inches long, what is the length of the painting?

A.
9 inches
B.
12 inches
C.
13 inches
D.
18 inches

Answer 1

12 inches

EXPLANATION

Let the length of the rectangular painting be
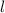`l` inches, then the width will be
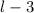`l-3` inches.

Since the diagonal is the hypotenuse, we can apply the Pythagoras Theorem to find the length.

`(l-3)^2+l^2=15^2`

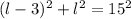
We expand to obtain;

`l^2-6l+9+l^2=225`

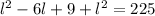
We simplify to obtain a quadratic equation in
`l`.

`2l^2-6l-216=0`

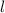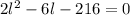
We divide through by 2 to obtain.

`l^2-3l-108=0`

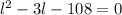
We factor to obtain

`l^2-12l+9l+108=0`

`l(l-12)+9(l-12)=0`

`(l-12)(l+9)=0`

`\Rightarrow l=12\:or\:l=-9=0`

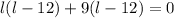
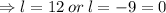
Since we are dealing with length we discard the negative value.

Hence the length is 12 inches

Answer 2

B. 12 inches

Explanation:

We know that the length of the rectangular painting is 3 inches longer than its width so we can write it as:

l = w + 3

and the diagonal is 15 inches long.

We can use use the Pythagoras Theorem to find the length of the painting.

(w)² + (w + 3)² = (15)²

w² + w² + 6w + 9 = 225

2w² + 6w - 216 = 0

w² + 3w - 108 = 0

(w + 12)(w - 9) = 0

w = -12 (ignore), w = 9

So, l = w + 3

l = 12

Therefore, the length of the rectangle is 12 inches.