Hello!
Slope-intercept form is y = mx + b. In this form, m is the slope and y is the y-intercept.
To find the slope, we need to use the slope formula. The slope formula is:
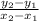 . We can assign the point (4, 6) to
. We can assign the point (4, 6) to
 and
and
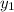 and (9, 16) to
and (9, 16) to
 and
and
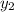 .
.
Now, we can find the slope:
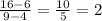
Next, we can find the y-intercept by substituting a point into the slope-intercept form with the slope as 2.
y = 2x + b (substitute a given point)
6 = 2(4) + b (simplify)
6 = 8 + b (subtract 8 from both sides)
-2 = b
Therefore, the equation of the line is y = 2x - 2.