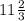Question

Please help asap!!!

1. ABCD is an isosceles trapezoid. m < A = 6x + 12 and m < d = 2x + 20. Angles B&C are congruent. What is the measure of angles B&C?


2. QRST is a rectangle. RS = 12k +5 and QT = 145. what is the value of 'k'?

Answer 1

∠ B = 123°, ∠ D = 57°

Explanation:

Each lower base angle is supplementary to the upper base angle on the same side.

∠ A and ∠ D are on the same side, then

6x + 12 + 2x + 20 = 180

8x + 32 = 180 ( subtract 32 from both sides )

8x = 148 ( divide both sides by 8 )

x = 18.5

Thus

∠ A = 6x + 12 = 6(18.5) + 12 = 111 + 12 = 123°

∠ D = 2x + 20 = 2(18.5) + 20 = 37 + 20 = 57°

The lower base angles and the upper base angles are congruent, so

∠ B = ∠ A = 123°

∠ C = ∠ D = 57°

Answer 2

Explanation:

#1). It could be good to provide us with the picture, because the answer depends from the angle we choose for letter A.

--- If angle A is acute, then m∠A = m∠D

and

6x + 12 = 2x + 20 ⇒ x = 2

m∠A = m∠D = 24°

m∠B = m∠C = 180° - 24° = 156°

--- If angle A is obtuse, then m∠A + m∠D = 180°

and

6x + 12 + 2x + 20 = 180 ⇒ x = 18.5

m∠A = m∠B = 6(18.5) + 12 = 123°

m∠D = m∠C = 2(18.5) + 20 = 57°

#2). 12k + 5 = 145

12k = 140

k =
`(35)/(3)` =
`11(2)/(3)`