Question

Is the following relation a function? (1 point) Graph of x equals y squared, which results in a horizontal parabola centered on the x axis, going through the origin, and opening to the right Yes No

Answer 1

The equation is not a function because in order for something to be a function you can't have 2 values of y for every x.

Answer 2

No, the given relation is not a function.

Explanation:

A relation is a function if there exist a unique output for each input.

The given relation is

`x=y^2`

It is a horizontal parabola centered on the x axis, going through the origin, and opening to the right.

Taking square root on both sides.

`\pm √(x)=y`

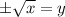
Here for each value of x, there exist more than one value of y. Therefore the given relation is not a function.

If a vertical line intersect the parabola more than once, then the relation is not a function.

From the below graph it is clear that the graph dose not pass the vertical line test. So, the given relation is not a function.