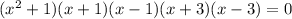Question

X^6-9x^4-x^2+9=0 please help me I don't understand this

Answer 1

Steps:

So firstly, I will be factoring by grouping. For this, factor x⁶ - 9x⁴ and -x² + 9 separately. Make sure that they have the same quantity on the inside of the parentheses:

`x^4(x^2-9)-1(x^2-9)=0`

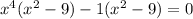
Now, you can rewrite the equation as:

`(x^4-1)(x^2-9)=0`

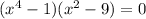
However, it's not completely factored. Next, we will apply the formula for the difference of squares, which is
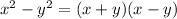`x^2-y^2=(x+y)(x-y)` . In this case:

`x^4-1=(x^2+1)(x^2-1)\\x^2-9=(x+3)(x-3)\\\\(x^2+1)(x^2-1)(x+3)(x-3)=0`

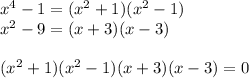
Next, we will apply the difference of squares once more with the second factor as such:

`x^2-1=(x+1)(x-1)\\\\(x^2+1)(x+1)(x-1)(x+3)(x-3)=0`

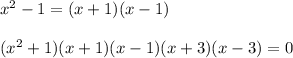
Answer:

The factored form of this equation is:
`(x^2+1)(x+1)(x-1)(x+3)(x-3)=0`