Question

What is the area of a rectangle with vertices at ​ (0, −4) ​, ​ (−1, −3) ​ , (2, 0) , and (3, −1) ? Enter your answer in the box.

Answer 1

We are given vertices of a rectangle (0, −4) ​, ​ (−1, −3) ​ , (2, 0) , and (3, −1).

Length is the distance between (0, −4) ​and ​ (−1, −3) points.

Width is distance between (−1, −3) ​ and (2, 0) points.

Computing length:

`\mathrm{Compute\:the\:distance\:between\:}\left(x_1,\:y_1\right),\:\left(x_2,\:y_2\right):\quad √(\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2)`

`=√(\left(-1-0\right)^2+\left(-3-\left(-4\right)\right)^2)`

`=√(2)`

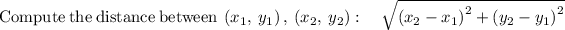
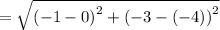
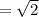
Computing Width :

`\mathrm{The\:distance\:between\:}\left(-1,\:-3\right)\mathrm{\:and\:}\left(2,\:0\right)\mathrm{\:is\:}`

`=√(\left(2-\left(-1\right)\right)^2+\left(0-\left(-3\right)\right)^2)`

`=3√(2)`

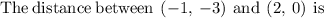
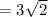
Area of the rectangle = Length × Width

=
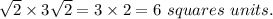`√(2) * 3√(2) = 3 * 2 = 6 \ squares \ units.`.