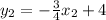Question

What is the equation of a line that passes through the point (8,-2) and is parallel to the line whose equations is 3x+4y=15

Answer 1

For this case we have:

When two lines are parallel, their slopes are equal.

Be a line of the form
`y = mx + b`

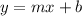
Where:

m is the slope

b is the cut point

If we have:
`3x + 4y = 15`

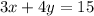
We can rewrite it as:

`4y = 15-3x`

`y = - \frac {3} {4} x + \frac {15} {4}`

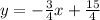
Thus, the slope of that line is given by
`m = - \frac {3} {4}`

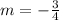
Since that line is parallel to the one we want to find, then
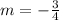`m = - \frac {3} {4}`is the same for both lines.

The equation of the line that we want to find follows the form:

`y_(2) = m_(2)x_(2) + b_(2)`

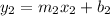
Where
`m_(2)= - \frac {3} {4}`

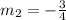
So, we have:

`y_(2) = - \frac {3} {4} x_(2) + b_(2)`

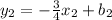
We have as data the point
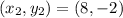`(x_(2), y_(2)) = (8, -2)` that passes through the line we want to find. Substituting the points we find the cut point
`b_(2)`:

`-2 = - \frac {3} {4} (8) + b_(2)`

`-2 = -6 + b_(2)\\b_(2) = -2 + 6\\b_(2) = 4`

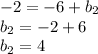
Thus, the equation of the requested line is given by:

`y_(2) = - \frac {3} {4} x_(2) + 4`

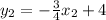
Answer:

`y_(2) = - \frac {3} {4} x_(2) + 4`