Question

Find the possible values of x.
the perimeter is less than or equal to 51 meters.

Answer 1

The value of x can be anything between 0 to 15

Solution-

Perimeter of a geometrical figure is the sum of lengths of all its sides.

So, the value of the perimeter of the given pentagon would be,

`=8+8+10+10+x\\\\=(36+x)\ meters`

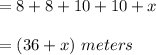
As given in the question that, the perimeter is less than or equal to 51 meters.

So,

`\Rightarrow 36+x\leq 51`

`\Rightarrow x\leq 15`

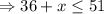
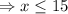
As the side length can not be negative, so x must be positive, i.e

`0<x\leq 15`