Question

The graph shows the distances traveled by two buses.

he equation y = 55x represents the distance, y, Bus A travels in x hrs.

Which equation could represent the distance traveled by Bus B?



y = 40x

y = 60x

y = 65x

y = 70x

Answer 1

y=40x

I've done the test and this was correct.

hope it helps :)

Answer 2

The equation that could represent the distance traveled by Bus B is y = 40x

Solution-

On abscissa time in hour is represented and on ordinate distance in km is represented.

The equation for Bus A is given by,

`y=55x`

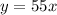
Comparing it with the general slope-intercept equation of straight line,

`y=mx+c`

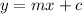
Where,

m = slope = 55

c = y-intercept = 0

We know that slope is measured as the tangent of the angle made by the line with positive x-axis measured in counter-clockwise direction.

Slope for Bus A = m₂ = tan θ₂

Slope for Bus B = m₁ = tan θ₁

From the properties of trigonometric function,

`\Rightarrow \tan \theta_1<\tan \theta_2\ \ \ \ \ \ (\because \theta_1<\theta_2)\\\\\Rightarrow m_1<m_2`

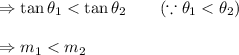
From the given option, only the first option has a straight line equation with slope less than m₂ or 55, which is 40.

Therefore, the equation that could represent the distance traveled by Bus B is y = 40x