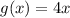Question

Which of the following is an odd function?

Answer 1

number 3 is an odd function

Answer 2

The odd function is D)
`g(x)=4x`

Explanation:

We need to check given options are odd function or not

Since, odd function is satisfy the f(-x) = -f(x)

Check for part A)
`g(x)=x^(2)`

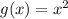
Replace x by - x in
`g(x)=x^(2)`

`g(-x)=(-x)^(2)`

`g(-x)=x^(2)`

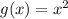
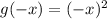
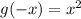
since, we can not write it as
`g(-x) = -g(x)`

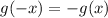
Hence, this is not an odd function

Check for part B)
`g(x)=5x-1`

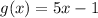
Replace x by - x in
`g(x)=5x-1`

`g(-x)=5(-x)-1`

`g(x)=-5x-1`

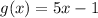
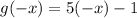
since, we can not write it as
`g(-x) = -g(x)`

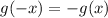
Hence, this is not an odd function

Check for part C)
`g(x)=3`

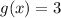
since, constant functions are even function

Hence, this is not an odd function

Check for part D)
`g(x)=4x`

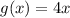
Replace x by - x in
`g(x)=4x`

`g(-x)=4(-x)`

`g(x)=-4x`

`g(x)=-g(x)`

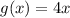
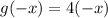
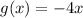
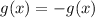
since, we can write it as
`g(-x) = -g(x)`

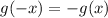
Hence, this is an odd function

Therefore, the odd function is D)
`g(x)=4x`