Question

A reaction of 55.1 g of Na and 31.1 g of Br2 yields 35.4 g of NaBr. What is the percent yield?

Answer 1

So,

% yield equals actual mass of product divided by theoretical mass of product times 100%.

1. First, write the balanced reaction.

`Na(s)+(1)/(2)Br_2(l) -->NaBr(s)`

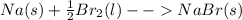
2. Since we are given the masses of both reactants, we need to do a Limiting Reactant calculation. Calculate the theoretical masses of product produced by each reactant, assuming excess of the other reactant.

a.
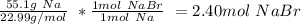
`(55.1g\ Na)/(22.99g/mol)\ *(1mol\ NaBr)/(1mol\ Na)\ =2.40mol\ NaBr`

b.
`(31.1g\ Br_2)/(159.8g/mol)\ *(1mol\ NaBr)/(0.5mol\ Br_2)\ =0.389mol\ NaBr`

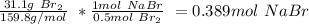
c. Bromine is the limiting reactant, and the theoretical mass of NaBr is:

`0.389mol\ NaBr\ *102.89g/mol\ =40.0 g\ NaBr`

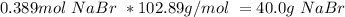
3. Now, we can calculate % yield.

`percent\ yield=(mass_(actual))/(mass_(theoretical))*100=(35.4g)/(40.0g)*100=88.5\ percent`

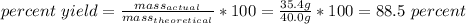
So, the percent yield is 88.5%.

Hope this helps!