Question

The point (- square-root 2/2, square-root2/2 is the point at which the terminal ray of angle theta intersects the unit circle. What are the values for the cosine and cotangent functions for angle theta?

Answer 1

Answer:a

Step-by-step explanation:

edge

Answer 2

Answer: The value of cosine is
`\frac{-√(2)} {2}` and the value of cotangent is -1.

Step-by-step explanation:

The given point is
`(\frac{-√(2)} {2}},\frac{√(2)} {2})`.

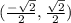Since the x coordinate is negative and y coordinate is positive so the point must be lies in second quadrant.

The distance of the point from the origin is,

`r=\sqrt{(\frac{-√(2)} {2}-0)^2+(\frac{√(2)} {2}-0)^2}`

`r=\sqrt{ (2)/(4)+(2)/(4)}`

`r=1`

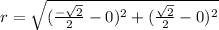
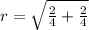
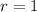
The given point is in the form of (a,b). So we get,

`a=\frac{-√(2)} {2}`

`b=\frac{√(2)} {2}`

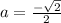
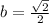
The formula for cosine,

`\cos \theta =(a)/(r)`

`\cos \theta =\frac{\frac{-√(2)} {2}}{1}}`

`\cos \theta =\frac{-√(2)} {2}}`

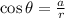
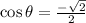
The formula for cotangent,

`\cot \theta =(a)/(b)`

`\cos \theta=\frac{\frac{-√(2)} {2}}{\frac{√(2)} {2}}`

`\cos \theta=-1`

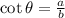
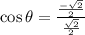
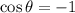
Therefore, the value of cosine is
`\frac{-√(2)} {2}` and the value of cotangent is -1.