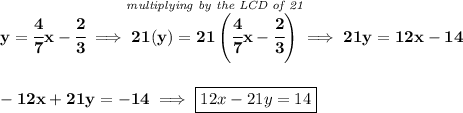Question

How would i convert slope intercept form y=4/7×-2/3 in standered form

Answer 1

keeping in mind that

standard form for a linear equation means

• all coefficients must be integers, no fractions

• only the constant on the right-hand-side

• all variables on the left-hand-side, sorted

• "x" must not have a negative coefficient

now, to do away with the denominators, we can just multiply both sides by the LCD of all fractions, in this case, those two fractions have an LCD of 21, so we'll multiply both sides by 21 to toss the denominators.

`\bf y=\cfrac{4}{7}x-\cfrac{2}{3}\implies \stackrel{\textit{multiplying by the LCD of 21}}{21(y)=21\left( \cfrac{4}{7}x-\cfrac{2}{3} \right)}\implies 21y=12x-14 \\\\\\ -12x+21y=-14\implies \boxed{12x-21y=14}`