Question

Please help me
10 points

Answer 1

Let us prove that angle 1 is complementary to angle 3 step by step.

1. We have been given that angle 1 is complementary to angle 2.

2. Since we know that complementary angles add up to 90 degrees, therefore, by the definition of complementary
`m\angle 1+ m\angle 2=90^(o)`.

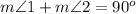3. We have been also given that line segment BD bisects
`\angle ADC`.

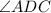4. By the definition of bisect
`\angle 2 \cong \angle 3`.

5. Angles are congruent if their measures, in degrees, are equal, therefore, by angle congruence postulate
`m\angle 2=m\angle 3`.

6.
`m\angle 1+ m\angle 2=90^(o)`

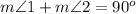
Upon substituting
`m\angle 2=m\angle 3` in above equation we will get,

`m\angle 1+ m\angle 3=90^(o)`

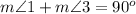
Therefore, by substitution property of equality
`m\angle 1+ m\angle 3=90^(o)` .

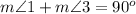Hence, proven that angle 1 is complementary to angle 3.