Question

The angle of the elevation from the top of a 95 Todd building into a hot air balloon in the sky and 76° if the horizontal distance between the building in a horrible Luna is 354 feet find the height of a hot air balloon

Answer 1

The height of the hot air balloon is 1514.54 feet approximately.

Explanation:

Consider the provided information.

The angle of elevation from the top of a 95-foot tall building to a hot air balloon in the sky is 76. If the horizontal distance between the building and the hot air balloon is 354 feet, find the height of the hot air balloon

The figure is shown below:

Let the height of the balloon is AD, the height of the building is BC.

Draw a line BE parallel to CD.

Therefore, AD=x+95, BC=95 and BE=CD=354 ft

Now in triangle
`ABE`.

`\tan\theta=\frac{\text{Opp}}{\text{Adj}}`

`\tan76^(\circ)=(AE)/(BE)`

`AE\approx 4.01* 354`

`AE\approx 1419.54`

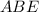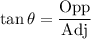
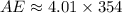
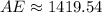
The height air balloon is 1419.54+95 =1514.54 ft

Hence, the height of the hot air balloon is 1514.54 feet approximately.