Answer-
Maria's monthly payment will be $376.91
Solution-
Maria is buying a car worth of $18,510, but she will finance only $13,000 of it with a 3-year loan at 2.8% APR.
We know that,
![\text{PV of annuity}=P[(1-(1+r)^(-n))/(r)]](https://img.qammunity.org/2019/formulas/mathematics/college/w5pyr6jen1rmdxycou5ktz8ihgjxwoqfgp.png)
Here,

Putting the values,
![\Rightarrow 13000=P[(1-(1+(2.8)/(1200))^(-36))/((2.8)/(1200))]](https://img.qammunity.org/2019/formulas/mathematics/college/fm4srtykgl0cj6vgnqmzffif108hnc8x43.png)
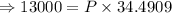
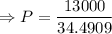
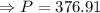
Therefore, her monthly payment will be $376.91