Question

Patrick is riding his bike on a rectangular path around his city. There is a rest area at each of the path's four corners. The city map shows the corners marked with coordinates of (–2, 2), (1, 2), (1, –2), and (–2, –2). Each unit represents 2.5 miles.

Answer 1

Question:

What is the perimeter of the path, in miles?

Answer:

35 miles

Explanation:

Given

Let the corners be ABCD

`A = (-2,2)`

`B = (1,2)`

`C = (1,-2)`

`D = (-2,-2)`

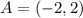
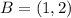
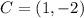
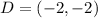
First, we calculate the perimeter of the corners in units.

This is done by calculating the distance between the corners.

Distance is calculated as:

`D = √((x_2 - x_1)^2 +(y_2 - y_1)^2 )`

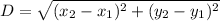
For AB:

`(x_1,y_1) = (-2,2)`

`(x_2,y_2) = (1,2)`

`D_1 = √((1 - (-2))^2 +(2 - 2)^2 )`

`D_1 = √((1 +2)^2 +(0)^2 )`

`D_1 = √(3^2)`

`D_1 = 3`

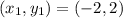
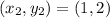
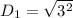
For BC

`(x_1,y_1) = (1,2)`

`(x_2,y_2) = (1,-2)`

`D_2 = √((1 - 1)^2 +(-2 - 2)^2 )`

`D_2 = √((0)^2 +(-4)^2 )`

`D_2 = √(16 )`

`D_2 = 4`

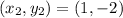
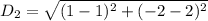
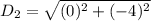
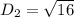
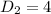
For CD:

`(x_1,y_1) = (1,-2)`

`(x_2,y_2) = (-2,-2)`

`D_3 = √((-2 - 1)^2 +(-2 - (-2))^2 )`

`D_3 = √((-3)^2 +(-2 +2)^2 )`

`D_3 = √(9 +0^2 )`

`D_3 = √(9 )`

`D_3 = 3`

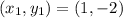
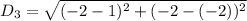
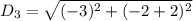
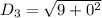
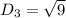
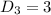
For DA

`(x_1,y_1) = (-2,-2)`

`(x_2,y_2) = (-2,2)`

`D_4 = √((-2 - (-2))^2 +(2 - (-2))^2 )`

`D_4 = √((-2 +2)^2 +(2 +2))^2 )`

`D_4 = √(0^2 +4^2 )`

`D_4 = √(4^2 )`

`D_4 = 4`

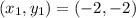
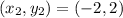
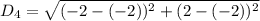
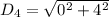
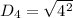
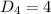
The perimeter P in units is:

`P = D_1 + D_2 + D_3 + D_4`

`P = 3+4+3+4`

`P = 14`

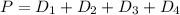
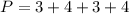
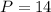
Given that:

`1\ units = 2.5\ miles`

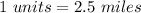
Multiply both sides by 14

`14 * 1\ units = 14 * 2.5\ miles`

`14 \ units = 35\ miles`

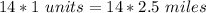
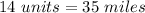
Hence, the perimeter is 35 miles