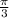Question

Q 2 PLEASE HELP ME FIGURE THIS OUT

Answer 1

Answer: IV, positive,
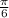`\frac{\pi} {6}`, - sec
`\frac{\pi} {6}`,
`(2√(3))/(3)`

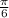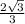
Explanation:

a) Look at the Unit Circle to see that
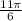`\frac{11\pi} {6}` = 330°, which is located in Quadrant IV.

b) The coordinate (cos θ, sin θ) for
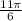`\frac{11\pi} {6}` is:
`(\frac{√(3)} {2},(-1)/(2))`

sec =
`(1)/(cos)` =
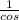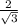`(2)/(√(3))` which is positive

c) Since the given angle is in Quadrant IV, which is closest to the x-axis at 360° = 2π, the reference angle can be found by subtracting the given angle
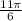`\frac{11\pi} {6}` from 2π:
`\frac{12\pi} {6}` -
`\frac{11\pi} {6}` =
`\frac{\pi} {6}`

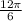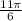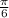
d) the reference angle is below the x-axis so the given angle is equal to the negative of the reference angle: - sec
`\frac{\pi} {6}`.

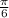e) sec
`\frac{11\pi} {6}` =
`(2)/(√(3))` =
`(2)/(√(3))*(√(3))/(√(3))` =
`(2√(3))/(3)`

***************************************************************************************

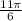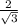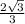
Answer:
`(18\pi)/(11)`, IV,
`\frac{4\pi} {11}`

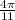
Explanation:

2π is one rotation. 2π =
`(22\pi)/(11)`

`(-26\pi)/(11)` +
`(22\pi)/(11)` =
`(-4\pi)/(11)`

`(-4\pi)/(11)` +
`(22\pi)/(11)` =
`(18\pi)/(11)`

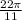
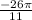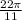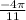
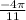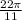Convert the radians into degrees to see which Quadrant it is in by setting up the proportion and cross multiplying:

`(\pi)/(180)`=
`(18\pi)/(11x)`

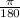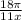
π(11x) = (180)18π

x =
`(180(18\pi)/(11\pi)`

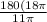
x = 295° which lies in Quadrant IV

Since the given angle is in Quadrant IV, which is closest to the x-axis at 360° = 2π, the reference angle can be found by subtracting the angle of least nonegative value
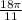`\frac{18\pi} {11}` from 2π:
`\frac{22\pi} {11}` -
`\frac{18\pi} {11}` =
`\frac{4\pi} {11}`

***************************************************************************************

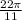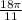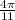
Answer:
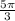`(5\pi)/(3)`, IV,
`\frac{4\pi} {11}`,
`\frac{\pi} {3}`

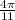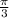
Explanation:

2π is one rotation. 2π =
`(6\pi)/(3)`

`(-13\pi)/(3)` +
`(6\pi)/(3)` =
`(-7\pi)/(3)`

`(-7\pi)/(3)` +
`(6\pi)/(3)` =
`(-\pi)/(3)`

`(-\pi)/(3)` +
`(6\pi)/(3)` =
`(5\pi)/(3)`

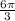
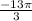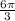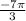
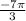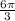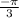
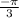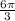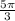
This is on the Unit Circle at 300°, which is located in Quadrant IV

Since the given angle is in Quadrant IV, which is closest to the x-axis at 360° = 2π, the reference angle can be found by subtracting the angle of least nonegative value
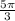`\frac{5\pi} {3}` from 2π:
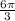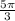`\frac{6\pi} {3}` -
`\frac{5\pi} {3}` =
`\frac{\pi} {3}`