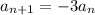Answer:
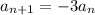
Explanation:
The recursive formula for geometric sequence is given by :-
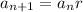 -----(1) , where r = common ratio and n=natural number .
-----(1) , where r = common ratio and n=natural number .
nth term of geometric sequence =
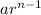
The given geometric sequence : 4,-12,36,-108...
First term =
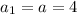
Second term =
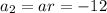
Also,
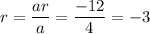
∴ r = -3
Put the value of r in (1) , we get the recursive formula for given geometric sequence as
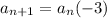
i.e.