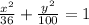
General equation is

Where (h,k) is the center
From the given equation h=0 and k=0
So center is (0,0)
compare the given equation with general equation
b^2 = 36 so b= 6
a^2 = 100 so a = 10
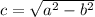
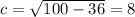
Vertices are (h, k+a) and (h, k-a)
We know h=0 , k=0 and a= 10
Vertices are (0,-10) and (0,10)
Foci are (h, k+c) and (h,k-c)
We know h=0 , k=0 and c=8
Foci are (0,-8) and (0,8)