Question

Evaluate the determinant for the following matrix: [- 5 1 1 4] A. –21 B. 19 C. –17 D. –24

Answer 1

-21

Explanation:

I assume this is a 2 by 2 matrix.

`\begin{bmatrix} -5 & 1\\1 & 4 \end{bmatrix}`

determinant = -5 * 4 - 1 * 1 = -20 - 1 = -21

Answer 2

Option A.

Explanation:

If a matrix is defined as

`A=\begin{bmatrix}a\:&\:b\:\\ c\:&\:d\:\end{bmatrix}\:`

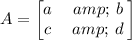
then its determinant is

`|A|=\:ad-bc`

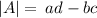
Let the given matrix is

`B=\begin{bmatrix}-5&1\\ \:1&4\end{bmatrix}`

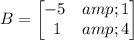
We need to find the determinant for the given matrix.

Using the above formula we get

`|B|=\left(-5\right)\cdot \:4-1\cdot \:1`

`|B|=-21`

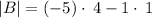
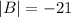
The determinant of given matrix is -21. Therefore, the correct option is A.