Question

A. Airplanes typically fly at an altitude of about 10,000 meters. At this altitude the atmospheric pressure is significantly less than it is at sea-level. Because of this, airplane cabins must be pressurized so that the people on board can continue to get enough oxygen. If a typical 747 has a volume of 28,000 m3 and is filled with air at 101.3 kPa on the ground, what is the new volume of air inside when the cabin is pressurized to 75 kPa once it is in flight? b. Twinkies are made up of 68% air. If you buy a Twinkie in the airport and take it with you on a plane ride, how would its size change during the flight?

Answer 1

The new volume of the air inside when the cabin is pressurized to 75 kPa is 37818.
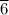`\overline 6` m³

Step-by-step explanation:

The given 747 airplane parameters are;

The volume of air in the airplane, V₁ = 28,000 m³

The pressure of the air on the ground, P₁ = 101.3 kPa

The pressure of the air once it is in flight, P₂ = 75 kPa

Let V₂ represent the new volume of the air inside when the cabin is pressurized to 75 kPa

Boyle's Law states that the pressure of a given mass of air is inversely proportional to its volume at constant temperature

Therefore, by Boyle's law, we have;

P ∝ 1/V and P₁·V₁ = P₂·V₂

Which gives;

V₂ = P₁·V₁/P₂

Substituting the known values, we get;

V₂ = 101.3 kPa × 28,000 m³/(75 kPa) = 37818.
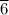`\overline 6` m³

The new volume of the air inside when the cabin is pressurized to 75 kPa = V₂ = 37818.
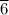`\overline 6` m³.