Question

Prove that opposite sides of a parallelogram are congruent. Be sure to create and name the appropriate geometric figures.

Answer 1

Theorem : Opposite sides of a parallelogram are congruent or equal.

Let us suppose a parallelogram ABCD.

Given:
`AB\parallel CD` and
`BC\parallel AD` (According to the definition of parallelogram)

We have to prove that: AB is congruent to CD and BC is congruent to AD.

Prove: let us take two triangles,
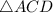`\bigtriangleup ACD` and
`\bigtriangleup ABC`

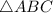
In these two triangles,
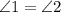`\angle1=\angle2` { By the definition of alternative interior angles}

Similarly,
`\angle4=\angle3`

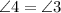
And, AC=AC (common segment)

By ASA,
`\bigtriangleup ACD \cong \bigtriangleup ABC`

thus By the property of congruent triangle, we can say that corresponding sides of
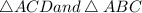`\bigtriangleup ACD and \bigtriangleup ABC` are also congruent.

Thus, AB is congruent to CD and BC is congruent to AD.