Answer:
The answer is

Explanation:
In order to determine the slope of the line, we have to know about how calculate a slope.
There are two ways of get a slope of a line:
- Knowing the linear equation and then we need to free the "y" variable (dependent variable). The coefficient of the "x" variable (independent variable) is the slope.
- From to two points that belong to the linear equation.
The formula of the second way is the next:
m=slope of the linear equation
P1=(x1,y1)=first pair of coordinate
P2=(x2,y2)=second pair of coordinate
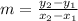
In this case, we have two points, so we use the formula:
P1=(-2,-1)
P2=(8,-3)
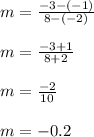
Finally, the slope of the line is
