Question

PLEASE HELP!!

2. Draw the image of ∆RST under the dilation with scale factor 5/3
and center of dilation (2, 2) − . Label the image ∆R′S′ T′. Show your work.

Answer 1

ΔRST is dilated by a factor of 1/3 with the center of dilation at the origin.The vertices of ΔRST, areR (3, 6)S (-3, 6)T (-6, -6)The vertices of the dilated image ΔR'S'T', areR' (1, 2)S' (-1,2)T' (-2, -2)The transformed image is shown in red color

Answer 2

From the given graph:

the coordinates of triangle RST are;

R= (2, 1),

S= (2,-2),

T= (-1,-2)

Given: Scale factor =
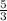`(5)/(3)` and center of dilation at (2,2)

The mapping rule for the dilation applied to the triangle as shown below:

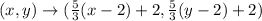`(x,y) \rightarrow ((5)/(3)(x-2)+2 , (5)/(3)(y-2)+2 )`; where k represents the scale factor i.e,
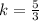`k=(5)/(3)` or we can write it as ;

For R=(2, 1)

The image R' =
`((5)/(3)(2-2)+2 , (5)/(3)(1-2)+2 )`

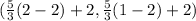
⇒ R'=
`(2, (1)/(3))`

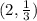
Similarly for S= (2, -2) and T= (-1,-2)

therefore, the image of S'=
`((5)/(3)(2-2)+2 , (5)/(3)(-2-2)+2 )`

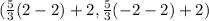
⇒ S'=
`(2, (-14)/(3))`

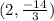
The image of T' =
`((5)/(3)(-1-2)+2 , (5)/(3)(-2-2)+2 )`

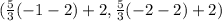
⇒T' =
`(-3, (-14)/(3))`

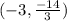
Now, labelling the image of triangle R'S'T' as shown in the figure given below