Answer:
Vertex → (2, 4)
Explanation:
Quadratic equation has been given as,
y = -x² + 4x
We rewrite this equation in the form of a function as,
f(x) = - x² + 4x
By comparing this equation with the standard quadratic equation,
y = ax² + bx + c
a = -1 and b = 4
Vertex of the parabola represented by this equation is given by
![[-(b)/(2a), f((-b)/(2a))]](https://img.qammunity.org/2022/formulas/mathematics/high-school/d4iyvi1c7zqc0dvps60hpn0uqncirv2wug.png)
x coordinate =
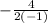
= 2
y-coordinate = f(2)
= - (2)² + 4(2)
= -4 + 8
= 4
Therefore, vertex of the given function is (2, 4)