For this case we have the following equation:
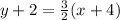
We can rewrite it in the way
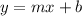
Where m is the slope and b represents the cut point.
So:
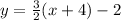
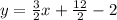
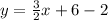
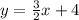
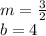
To graph, we look for two points through which the line passes:
Doing
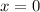 we have:
we have:
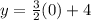
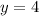
So, we have the point:
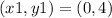
By doing
 we have:
we have:
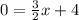
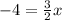

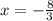
So, we have the point:
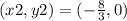
We locate
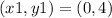 and
and
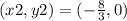 in a coordinate plane and the graph is attached.
in a coordinate plane and the graph is attached.
Answer:
See attached image