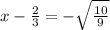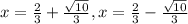Answer:
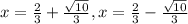
Explanation:
Multiplying the coefficient of x by the constant to get:
3 x (-2) = -6
Find factors of -6 that equal the middle term -4.
Since, no such factors can be found so we can solve the equation by completing the square.
To complete the square, divide the equation by the coefficient of x^2 which is 3 to get:
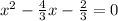
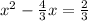
Now divide the coefficient of x by 2 and add the square of the result to both sides of the equation:
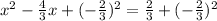
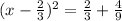
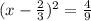
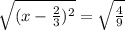
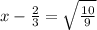 ,
,