For this case we have a system of two linear equations with two unknowns. whose variables are given by x and y respectively.
To solve the system by the linear combination method we follow the following steps:
1st step:
We multiply the first equation by 5:
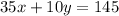
2nd step:
We multiply the second equation by 2:
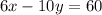
3rd step:
We add the equations:
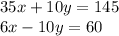
Thus, we obtain the following:

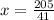
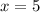
4th step:
We substitute
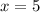 in any of the equations:
in any of the equations:


Thus, the values of the variables are
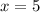 and
and
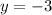 .
.
Answer:
The values are:
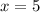 and
and
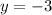 .
.