Question

Hurry!

Consider the function f(x)=3x-1 (over)x+4

(a) At which value of x will the function not have a solution? Explain your answer.

(b) if g(x) is a vertical shift of 4 units from f(x), write the function of g(x).How does the graph of g(x) compare to the graph of f(x) ? Explain the function you wrote.

(c) What is the value of x when g(x) 8? Show your work

Answer 1

Ans(a):

Given function is
`f(x)=(3x-1)/(x+4)`

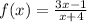
we know that any rational function is not defined when denominator is 0 so that means denominator x+4 can't be 0

so let's solve

x+4≠0 for x

x≠0-4

x≠-4

Hence at x=4, function can't have solution.

Ans(b):

We know that vertical shift occurs when we add something on the right side of function so vertical shift by 4 units means add 4 to f(x)

so we get:

g(x)=f(x)+4

`g(x)=(3x-1)/(x+4)+4`

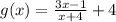
We may simplify this equation but that is not compulsory.

Comparision:

Graph of g(x) will be just 4 unit upward than graph of f(x).

Ans(c):

To find value of x when g(x)=8, just plug g(x)=8 in previous equation

`8=(3x-1)/(x+4)+4`

`8-4=(3x-1)/(x+4)`

`4=(3x-1)/(x+4)`

`4(x+4)=(3x-1)`

`4x+16=3x-1`

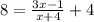
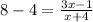
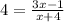
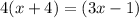
4x-3x=-1-16

x=-17

Hence final answer is x=-17