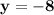Answer:
The equation of the line that passes through the points (7,-8) and (2, -8) is
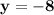
Explanation:
We need to write the equation of the line that passes through the points (7,-8) and (2, -8).
We need to write answer in fully reduced point-slope form, unless it is a vertical or horizontal line.
The general equation of point-slope form is:

where m is slope of the line.
To find the slope, we can use formula:
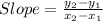
We have
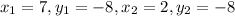
Putting values and finding slope:
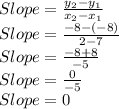
So, we find the slope : m = 0
Now, using the point (7,-8) and slope m =0, the required equation is:
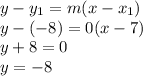
So, the equation of the line that passes through the points (7,-8) and (2, -8) is