Answer:
90.2 ft.
Explanation:
We can divided both numbers to find the answer, and that can be done rapidly:
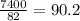
However, 7400 and 82 are too different number to use them as compatible numbers, but we can simplify the fraction step by step, we can divide each part by 2, which would give
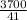 , and we will have minor numbers, making easier the division.
, and we will have minor numbers, making easier the division.
In addition, this division is allowed because it's based on the definition of a rectangle area, which is:
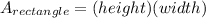
The problem gives us the total are and one side, we just need to replace values and isolate the side missing:

Therefore, the width of the hall is 90.2 ft.