Answer:
The value of b is 5.
Explanation:
Given: Line AB passes through points A(-6,6) and B(12,3).
Slope- intercept form:-The equation of a line with slope m and making an intercept b on y -axis is y = mx + b.
Since the line passes through two points therefore, we can use the Two Point Form formula:
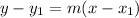 ; where m is the slope.
; where m is the slope.
or Slope

First find the value of m using the points A(-6,6) and B(12,3) ;
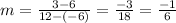
Now, the equation of line AB :-
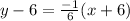 or
or
 or
or
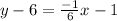 or
or
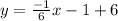
Simplify:
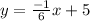
Comparing above equation with the general equation of line i.e, y = mx+b , we get;
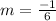 and b= 5
and b= 5
Therefore, the value y-intercept (b) = 5