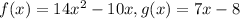
We need to find (fog)(x). Question says composition functions
(fog)(9)= f (g (9))
First we find g(9)
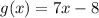
g(9)= 7(9) - 8 = 55
(fog)(9)= f (g (9))
Plug in 55 for g(9)
(fog)(9)= f (g (9)) = f(55)
Now we plug in 55 for x in f(x) and find f(55)

so (fog)(9) = 41,800