Question

Find equation of line in slope-intercept from having a slope 2/3 and passing through the point (-6, 4)

Answer 1

y=2/3x+8

Explanation:

y=mx+b

2/3=m

Answer 2

For this case we have the following data:

Slope,
`m = (2)/(3)`

`(x, y) = (- 6,4)`

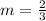
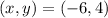
By definition, the equation of the slope-intercept form is given by:

`y = mx + b`

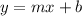
We must find the cut point b, for this we substitute the given point and the slope in the equation:

`4 =(2)/(3)(-6) + b`

`4 =-(12)/(3)+ b`

`4 = -4 + b\\4 + 4 = b\\b = 8`

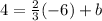
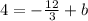
Thus, the equation is given by:

`y =(2)/(3)x + 8`

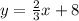
Now, we look for the points of intersection with the x and y axes respectively:

Point of intersection with the y axis:

We do
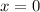`x = 0` and substitute in the equation found:

`y =(2)/(3)(0) +8\\y = 8`

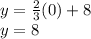
Thus, the point of intersection with the y-axis is (0,8).

Point of intersection with the x axis:

We make
`y = 0`and substitute in the equation found:

`0 =(2)/(3)x+ 8`

Clear x:

`(2)/(3)x= -8\\ 2x = -8 * 3`

`x = -(24)/(2)\\x = -12`

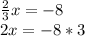
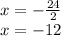
Thus, the point of intersection with the x-axis is (-12.0).

Answer:

`y =(2)/(3)x + 8`

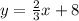
See attached image