Question

Find the derivative

Answer 1

This is loaded question, but let me take a stab at it:

`(d)/(dx) \cot^(-1) √(x-1) = (d\cot u)/(du)\cdot (du)/(dx)`

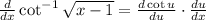
with
`u=√(x-1)`

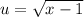
Start with the first part of the chain rule term:

`y = \cot^(-1) u\\(dy)/(du) = (d \cot^(-1)u)/(du)`

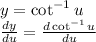
because cot^-1 is inverse to cot we also know that

`\cot y = u`

and use it in the earlier equation:

`(dy)/(du) = (d \cot^(-1)u)/(du)\\(1)/((d\cot y)/(dy)) = (d \cot^(-1)u)/(du)\\-\sin^2y=(d \cot^(-1)u)/(du)\\-(1)/(\cot^2 y +1) = (d \cot^(-1)u)/(du)\\-(1)/(u^2 +1) = (d \cot^(-1)u)/(du)`

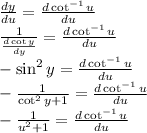
Now, the second term of the chain rule:

`(du)/(dx)=(d√(x-1))/(dx) = (1)/(2√(x-1))`

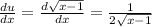
Putting it together:

`(d \cot^(-1)u)/(du)\cdot(du)/(dx) = -(1)/(x-1 +1)\cdot(1)/(2√(x-1)) = - (1)/(2x√(x-1))`

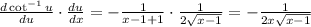
and that is the final derivative of your expression.