Question

Construct a function whose reflection in the line of y=x is itself. State the symmetries of the function.

Answer 1

ANSWER TO PART A

The mapping for the reflection in the line
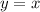`y=x`, is given by

`(x,y)\rightarrow (y,x)`.

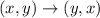That is the coordinates swap position .

The only way we can construct a function
`f(x)`, such that;

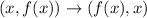`(x, f(x))\rightarrow (f(x),x)` are equal is when

`f(x)=x`.

So that when
`x=a, f(a)=a` .

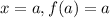The mapping then becomes

`(a,a)\rightarrow (a,a)`.

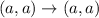Therefore the function,
`f(x)=x` is the function whose reflection in the line

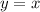`y=x` is itself.

ANSWER TO PART B

The function is symmetrical with respect to the origin. That is to say the function is an odd function.

A function is symmetric with respect to the origin, if it satisfies the condition,

`f(-x)=-f(x)`

For instance,

`f(a)=a`

`f(-a)=-a`

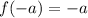
Since

`f(-a)=-a=-f(a)`

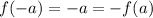
We say the function is symmetric with respect to the origin.